Filtered Rings

Definition.

Let A be a ring. A filtration on A is a sequence of additive subgroups

$A = A_0 \supseteq A_1 \supseteq A_2 \supseteq \ldots$

such that $A_i A_j \subseteq A_{i+j}$ for any $i, j\ge 0$ . A filtered ring is a ring with a designated filtration.

Note

Since $A\cdot A_i = A_0 \cdot A_i \subseteq A_i$ , in fact each $A_i$ is an ideal of A.

Examples

1. If $A = \oplus_{i=0}^\infty A_i$ is a grading, we can form a filtration by taking $n \mapsto A_n \oplus A_{n+1} \oplus \ldots$ (where $A_n, A_{n+1}, \ldots$ refers to the grading).

2. Let $\mathfrak a\subseteq A$ be an ideal. The $\mathfrak a$ –adic filtration is given by $A_i = \mathfrak a^i$ . E.g. we can take $A = \mathbb Z$ and $\mathfrak a = 2\mathbb Z$ , then $A_i$ is the set of integers divisible by $2^i$ . More generally, in a dvr with uniformizer $\pi$ , we can take $A_i = (\pi^i)$ .

Definition.

Suppose A is a filtered ring. A filtration on an A-module M is a sequence of additive subgroups

$M = M_0 \supseteq M_1 \supseteq M_2 \supseteq \ldots$

such that $A_i M_j \subseteq M_{i+j}$ for any $i, j\ge 0$ . A filtered module is a module with a designated filtration.

Note

Since $A\cdot M_i = A_0 M_i \subseteq M_i$ , each $M_i$ is an A-submodule of M.

Also, we need a fixed filtration on the base ring A before we can talk about filtrations on A-modules.

Example

Again, for an ideal $\mathfrak a \subseteq A$ , we obtain the $\mathfrak a$ –adic filtration of M, where $M_i = \mathfrak a^i M$ .

blue-lin

Induced Filtrations

For the rest of this article, A denotes a filtered ring.

Definition.

Let M, N be filtered A-modules. A linear map $f:M\to N$ is said to be filtered if $f(M_i) \subseteq N_i$ for each i.

We also have:

Definition.

Let $f:M\to N$ be a linear map of A-modules.

If $(M_i)$ is a filtration of M, the induced filtration on N via f is given by $N_i = f(M_i)$ .

If $(N_i)$ is a filtration of N, the induced filtration on M via f is given by $M_i = f^{-1}(N_i)$ .

Note

Let us show that the induced filtrations are legitimate. In the first case,

$A_i N_j = A_i f(M_j) = f(A_i M_j) \subseteq f(M_{i+j}) = N_{i+j}$ .

And in the second,

$f(A_i M_j) = A_i\cdot f(M_j) \subseteq A_i N_j \subseteq N_{i+j} \implies A_i M_j \subseteq M_{i+j}.$

In particular, if M is a filtered A-module and $N\subseteq M$ is a submodule, the induced filtrations on N and M/N are given by:

$N_i = M_i \cap N, \quad (M/N)_i = (M_i + N)/N.$

So far everything is natural, but beneath all this a danger lurks.

warning If $f:M\to N$ is a filtered A-linear map, then $M/\mathrm{ker} f$ and $\mathrm{im }f$ have induced filtrations via quotient module of M and submodule of N. Although $M/\mathrm{ker } f \cong \mathrm{im }f$ as A-modules, it is not an isomorphism in the category of filtered A-modules!

Let us write everything out explicitly. The filtration on the LHS and RHS are given respectively by

$(M / \mathrm{ker } f)_i = (M_i + \mathrm{ker } f)/\mathrm{ker } f, \quad (\mathrm{im } f)_i = (\mathrm{im} f) \cap N_i$

which gives

$(M_i + \mathrm{ker } f)/\mathrm{ker } f \cong M_i / (M_i \cap \mathrm{ker } f) = M_i / \mathrm{ker} (f|_{M_i}) \cong \mathrm{im} (f|_{M_i})$

which is not isomorphic to $(\mathrm{im} f) \cap N_i$ in general.

blue-lin

Completion

Definition.

Let M be a filtered A-module, the completion of M is the following (inverse) limit of A-modules:

$\hat M := \varprojlim M/M_n.$

By the description of inverse limits in the category of A-modules, $\hat M$ comprises of the set of all $(\ldots, x_n, \ldots, x_2, x_1) \in \prod_{i=1}^\infty (M/M_i)$ such that for each i, $x_{i+1}$ maps to $x_i$ under the canonical map $M/M_{i+1}\to M/M_i$ .

The canonical maps $M\to M/M_n$ induce an A-linear map $M \to \hat M$ by the universal property of inverse limits. This can be described as follows: for each $m\in M$ , let $m_i$ be its image in $M/M_i$ ; then the map takes $(m \in M) \mapsto (\ldots, m_n, \ldots, m_2, m_1) \in \hat M$ . From this we see that:

Lemma 1.

The map $i:M\to \hat M$ is injective if and only if $\cap_n M_n = 0$ , in which case we say the filtration is Hausdorff.

Next, by setting M = A, we also have $\hat A = \varprojlim A/A_n$ . Although we defined $\hat A$ as an A-module, one sees by the explicit construction that $\hat A$ has a ring structure. To be specific,

$(a_n)_{n=1}^\infty, (b_n)_{n=1}^\infty \in \hat A \implies (a_n) \times (b_n) := (a_n b_n)_{n=1}^\infty.$

Exercise A

1. Prove that $\hat M$ has a canonical structure as an $\hat A$ -module.

2. Prove that $\hat A$ is the inverse limit of $A/A_n$ in the category of rings. In particular, the canonical map $i : A\to \hat A$ is a ring homomorphism.

3. Prove that if the filtration on M is Hausdorff, we can define a metric on M via:

$d(x, y) := 2^{-|x-y|}$ , where $|z| := \sup\{ n : z\in M_n\},$

such that the collection of all cosets $\{ m + M_n : m\in M, n \ge 0\}$ forms a basis for the resulting topology. In fact, d is an ultrametric.

blue-lin

Limits in Completion

The completion $\hat M$ enables us to take limits of infinite sequences and sums of infinite series in modules.

Definition.

Let $m_1, m_2, \ldots \in M$ be a sequence in a filtered module M. We say the sequence is Cauchy if: for any i, there exists n such that

$m_n \equiv m_{n+1} \equiv m_{n+2} \equiv \ldots \pmod {M_i}$ .

The astute reader would note that if the filtration is Hausdorff, then $(m_n)$ is Cauchy here if and only if it is Cauchy with respect to the metric of exercise A.3.

Definition.

The limit of a Cauchy sequence $m_1, m_2, \ldots \in M$ is defined as follows. For each i, pick n such that $m_n \equiv m_{n+1} \equiv \ldots$ modulo $M_i$ , with image $y_i \in M/M_i$ . Now define:

$\lim_{n\to\infty} m_n := (\ldots, y_n, \ldots, y_2, y_1) \in \hat M$ .

From the definition, it is clear that if $(m_n), (m_n')$ (resp. $(a_n)$ ) are Cauchy sequences in M (resp. in A), then $(m_n + m_n')$ and $(a_n m_n)$ are also Cauchy and we have

$\begin{aligned}\lim_{n\to\infty} (m_n + m_n') &= (\lim_{n\to\infty} m_n) + (\lim_{n\to\infty} m_n') \in \hat M, \\ \lim_{n\to\infty} (a_n m_n) &= (\lim_{n\to\infty} a_n) (\lim_{n\to\infty} m_n) \in \hat M.\end{aligned}$

Example: 𝔪-adic Completion

Pick a ring A with maximal ideal $\mathfrak m$ ; we will take the $\mathfrak m$ -adic filtration $A_n = \mathfrak m^n$ . Given a sequence $x_i \in A_i$ for $i=0, 1, \ldots$ , let

$y_i := x_0 + \ldots + x_{i-1} \pmod {\mathfrak m^i}$ , an element of $A/A_i$ .

Then $(y_i)$ is a Cauchy sequence in A and we write:

$\sum_{i=0}^\infty x_i := \lim_{n\to\infty} y_n \in \hat A$ .

Arithmetic Example.

Let $A = \mathbb Z$ and $A_n = p^n \mathbb Z$ for a prime p. The completion $\hat A$ is called the ring of p-adic integers and denoted by $\mathbb Z_p$ . Let us take p = 2 and the element:

$y = 1 + 2^2 + 2^4 + 2^6 + \ldots \in \mathbb Z_2.$

Then $3y = 3 + 3(2^2) + 3(2^4) + \ldots$ is congruent to -1 modulo any $2^n$ . Thus $y = -\frac 1 3$ . In general, an element of $\mathbb Z_p$ can be regarded as having an infinite base-p expansion. Thus the above $y \in \mathbb Z_2$ would have base-2 expansion $(\ldots 1010101)_2$ . One easily checks that three times this value is $(\ldots 1111)_2$ , which is -1.

Geometric Example

Let A be a ring, $B = A[X, Y]$ and $B_n = \mathfrak m^n$ where $\mathfrak m = (X, Y)$ . Again, we can take the infinite sum $\alpha = 1 + XY + (XY)^2 + \ldots$ and check that $\alpha(1-XY) = 1$ . Note that $\hat B \cong A[[X,Y]]$ , the ring of formal power series with coefficients in A.

Definition.

Let A be any ring. A formal power series in X with coefficients in A is an expression

$f(X) = a_0 + a_1 X + a_2 X^2 + \ldots$ , where $a_i \in A$ .

Unlike polynomials, we allow infinitely many $a_i$ to be non-zero. Addition and multiplication of formal power series are defined as follows. For $f(X) = \sum_{i=0}^\infty a_n X^n$ and $g(X) = \sum_{j=0}^\infty b_n X^m$ ,

$\begin{aligned} f(X) + g(X) &=(a_0 + b_0) + (a_1 + b_1)X + (a_2 + b_2)X^2 + \ldots \\ f(X)\times g(X) &= (a_0 b_0) + (a_0 b_1 + a_1 b_0)X + (a_0 b_2 + a_1 b_1 + a_2 b_0)X^2 + \ldots \end{aligned}$

This gives a ring structure on the set $A[[X]]$ of formal power series. To define rings of formal power series in multiple variables, we set recursively

$A[[X_1, \ldots, X_n]] := (A[[X_1, \ldots, X_{n-1}]])[[X_n]]].$

As another example, let us take the $\mathfrak m$ -adic completion for $A = \mathbb C[X, Y]/(Y^2 - X^3 + X)$ and $\mathfrak m = (X, Y)$ . We will prove later that

$\hat A \cong \mathbb C[[X, Y]]/(Y^2 - X^3 + X)$

and that the map $\mathbb C[[Y]] \to \hat A$ is an isomorphism. Geometrically, this means when we project $E: Y^2 = X^3 - X$ to the Y-axis, the map is locally invertible at the origin.

completion_diagram_v1

[ Image edited from GeoGebra plot. ]

warning Functorially, the ring $A[[X, Y]]$ behaves quite differently from $A[X, Y]$ , because as an A-module, it is a countably infinite direct product of copies of A, unlike $A[X, Y]$ which is a direct sum. If we follow the earlier guideline, it is (for example) generally false that $B \otimes_A A[[X, Y]] \cong B[[X, Y]]$ for any A-algebra B.

Exercise B

1. Prove that the canonical map $\mathbb C[[X]] \to \mathbb C[[X, Y]]/(Y^2 - X^3 + X)$ is not an isomorphism.

2. Let A be a filtered ring. Prove that if $f(X) \in A[[X]]$ is $a_0 + a_1 X + a_2 X^2 + \ldots$ , then f defines a map

$A_1 \to \hat A, \quad f(\alpha) := \sum_{n=0}^\infty a_n \alpha^n \in \hat A.$

Prove that if we fix $\alpha \in A_1$ , we get a ring homomorphism $A[[X]] \to \hat A$ , $f\mapsto f(\alpha)$ .

blue-lin

5 Responses to Commutative Algebra 54

Vanya says:

August 3, 2020 at 6:18 am

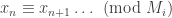In the first definition in the section “Limits in completion” it is mentioned that “Let $m_1, m_2, \ldots \in M$ , but in the congruences immediately following that sentence $x_n \equiv x_{n+1} \dots \pmod{M_i}$ .

Vanya says:

August 3, 2020 at 6:45 am

The scalar multiplication just before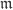 $\mathfrak{m}$ -adic completion has a typo.

- limsup says:
  
  August 3, 2020 at 3:48 pm
  
  Thanks! Corrected this error and the earlier one.
  
Vanya says:

August 3, 2020 at 12:00 pm

What does “locally invertible at the origin” in the diagram mean?

- limsup says:
  
  August 3, 2020 at 3:53 pm
  
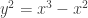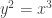  This one should be regarded as just a form of geometric intuition. If we “zoom in” the graph at the origin, eventually it resembles a straight line. For a non-example, if we “zoom in” the graph of $y^2 = x^3 - x^2$ or $y^2 = x^3$ at the origin, you will see a singularity there.
  
  This sounds familiar: you might have seen this earlier for localization, but the completion takes the intuition one step further. For example, the completion of $A = \mathbb C[X, Y]/(Y^2 - X^3 + X)$ at the origin is in fact isomorphic to $\mathbb C[[Y]]$ yet the localization of $A$ at the origin is not the localization of a polynomial ring.