Catenary Rings

Let us look at prime chains in greater detail.

Definition.

Let $\mathfrak p_0 \subsetneq \ldots \subsetneq \mathfrak p_d$ be a chain of prime ideals of a ring A. We say the chain is

saturated if for any prime ideal $\mathfrak q$ of A,

$\mathfrak p_i \subseteq \mathfrak q \subseteq \mathfrak p_{i+1} \implies \mathfrak q = \mathfrak p_i \text{ or } \mathfrak q = \mathfrak p_{i+1}$ ;

maximal if it is saturated, $\mathfrak p_0$ is a minimal prime and $\mathfrak p_d$ is a maximal ideal.

In words, saturated means we cannot insert a prime ideal between any two consecutive terms of a chain; maximal means in addition, we cannot extend the chain further in either direction.

Definition.

A ring is said to be catenary if, for all primes $\mathfrak p, \mathfrak q \subset A$ , all saturated prime chains with fixed ends

$\mathfrak p = \mathfrak p_0 \subsetneq \mathfrak p_1 \subsetneq \ldots \subsetneq \mathfrak p_d = \mathfrak q$

have the same length d.

catenary_spec

Note

If A is a catenary ring, so is any of its quotient $A/\mathfrak a$ or localization $S^{-1}A$ since the resulting spectrum is a subspace of Spec A.

blue-lin

Clarifications

Since dimension theory can be rather tricky, we will spend some time to clarify some of the above concepts.

1. On Catenary Rings

Catenary rings A are extremely common. In fact, it takes quite a bit of effort to construct a noetherian ring which is not catenary and such examples are usually pathological. See this article from Stacks project for an explicit construction by Nagata.

2. Maximal Prime Chains in Catenary Rings

warning However, even in a catenary ring A, not all maximal prime chains may be of the same length. The reason is rather simple: A can have multiple minimal prime ideals and multiple maximal ideals. The catenary condition does not specify that every saturated prime chain from every minimal prime to every maximal prime must be of the same length.

E.g. in the following Spec A, the blue prime chains may be longer than the green ones.

catenary_spec_v2

With multiple minimal primes, it is easy to construct catenary rings with maximal prime chains of different lengths. For example if $B = A_1 \times A_2$ , the set of prime chains of B is the union of that of $A_1$ and of $A_2$ . So if we pick $A_1, A_2$ of different dimensions like $B = \mathbb C \times \mathbb C[X]$ we get maximal prime chains of different lengths:

$0 \times \mathbb C[X], \qquad \mathbb C \times 0 \subset \mathbb C \times \mathbb (X)$ .

3. Catenary Rings Which Are Integral Domains

What if we specify that A is an integral domain? This ensures that there is a unique minimal prime: 0 itself. There remains the possibility of multiple maximal ideals. When A is a finitely generated algebra over a field, indeed all maximal prime chains have the same length (to be proven later).

In the general case, we have the following counter-example $A = \mathbb Z_{\mathfrak p}[X]$ where $\mathfrak p = 2\mathbb Z$ . This has maximal prime chains

$0 \subset (2X - 1), \qquad 0 \subset (2) \subset (2, X)$

of different lengths.

4. Dimension of Noetherian Rings

If A is a noetherian ring, every chain of ideals of A must eventually terminate so we cannot have infinite prime chains. But this does not mean $\dim A$ must be finite, for A may have maximal prime chains of arbitrary length. For example, Nagata constructed a noetherian ring A whose spectrum looks like the following,

nagata_infinite_dim

where the i-th column is a maximal prime chain of length i-1. In particular, $dim A = \infty$ .

Exercise A

1. Decide if each of the following is true or false.

If A is a catenary ring which is a local integral domain, then all maximal prime chains have the same length.
If A is a catenary ring which is a local ring, then all maximal prime chains have the same length.

2. Let A be a noetherian domain.

Prove that if the prime ideal $\mathfrak p \ne 0$ of A is principal, then it is a minimal non-zero prime.
Prove that if A is a UFD, any minimal non-zero prime ideal is principal.

3. Prove that the two prime chains in example 3 are maximal.

4. Prove that any maximal prime chain of $\mathbb Z[X]$ has length 2. In particular, $\mathbb Z[X]$ is catenary and hence the ring in example 3 is catenary since it is a localization of $\mathbb Z[X]$ .

blue-lin

More Consequences of NNT

Now we consider finitely generated k-algebras where k is a field.

Proposition 1.

Let $A = k[X_1, \ldots, X_n]$ . All maximal prime chains of A have the same length n.

Note

Although we already have dim A = n, this is a significantly stronger result.

Proof

The proof is by induction on n. We may assume $n\ge 1$ .

Note that 0 is a prime ideal of A. Let $\mathfrak p$ be a minimal non-zero prime and $B = A/\mathfrak p$ . By exercise A.2 above $\mathfrak p =(f)$ since A is a UFD. By exercise B here, B has transcendence degree n – 1 so by induction hypothesis any maximal prime chain of B has length n – 1. Since $\mathfrak p$ is an arbitrary minimal non-zero prime this shows that any maximal prime chain of A has length n. ♦

With this as a base, we may now prove the more general result.

Proposition 2.

Let A be a finitely generated k-algebra which is also an integral domain. All maximal prime chains of A have the same length n.

Note

We must have $n = \dim A = \mathrm{trdeg} A/k$ .

Proof

Since A is finitely generated we can write it as $A\cong k[X_1, \ldots, X_m]/\mathfrak p$ for a prime ideal $\mathfrak p \subset k[X_1, \ldots, X_m]$ . Fix a saturated prime chain of $k[X_1, \ldots, X_m]$ which starts at 0 and ends at $\mathfrak p$ ; let j be its length.

Now suppose we pick any maximal prime chain of A; let l be its length. The chain must start at 0 and end at a maximal ideal, which corresponds to a prime chain of $k[X_1, \ldots, X_m]$ starting at $\mathfrak p$ and ending at a maximal ideal (containing $\mathfrak p$ ). Appending the prior chain, we thus obtain a maximal prime chain of $k[X_1, \ldots, X_m]$ . By proposition 1, we must have $j + l = m$ so $l = m-j$ is independent of the maximal prime chain of A we chose. ♦

blue-lin

Height of an Ideal

Definition.

Let $\mathfrak p \subset A$ be a prime ideal. The height of $\mathfrak p$ , denoted by $\mathrm{ht} \mathfrak p$ , is the supremum of the lengths of prime chains ending at $\mathfrak p$ .

In other words $\mathrm{ht} \mathfrak p = \dim A_{\mathfrak p}$ . Since $\dim A/\mathfrak p$ is the supremum of the lengths of prime chains starting from $\mathfrak p$ , it follows that we always have

$\mathrm{ht} \mathfrak p + \dim A/\mathfrak p \le \dim A.$

We can also define the height of any ideal.

Definition.

Let $\mathfrak a\subseteq A$ be an ideal. The height of $\mathfrak a$ , denoted by $\mathrm{ht}\mathfrak a$ , is the infimum of $\mathrm{ht} \mathfrak p$ over all primes $\mathfrak p \supseteq \mathfrak a$ .

Note

Here is the intuition behind the definition. Note that $\dim A/\mathfrak a$ is the supremum of $\dim A/\mathfrak p$ over all $\mathfrak p \supseteq \mathfrak a$ . Thus to “compensate” for taking the supremum here, we let $\mathrm{ht} \mathfrak a$ take the infimum of $\mathrm{ht}\mathfrak p$ over all $\mathfrak p\supseteq \mathfrak a$ .

From the previous section, we obtain:

Corollary 1.

If A is a finitely generated k-algebra and also an integral domain, then for any prime ideal $\mathfrak p\subset A$ we have

$\mathrm{ht} \mathfrak p + \dim A/\mathfrak p = \dim A.$

In particular, A is a catenary ring.

More generally we have:

Corollary 2.

Any finitely generated algebra A over a field is catenary.

Hence so is any localization of such an algebra.

Proof

A must be a quotient of some $k[X_1, \ldots, X_m]$ so it is catenary. ♦

blue-lin

2 Responses to Commutative Algebra 43

Vanya says:

May 23, 2020 at 1:36 pm

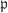Is it not required to add the following at the end of the first paragraph of the proof of Proposition 2? : The local ring $k[X_1, \ldots, X_m]_{\mathfrak{p}}$ is a local domain so all maximal chains have the same length. Therefore the corresponding saturated prime chains which starts at $(0)$ and ends at $\mathfrak{p}$ have the same length.

- limsup says:
  
  May 23, 2020 at 2:40 pm
  
  Actually I don’t need to add that statement, although it’s true. By the end of the proof, it also follows that all saturated prime chains starting at $(0)$ and ending at $\mathfrak p$ are of the same length.

Commutative Algebra 43

Catenary Rings